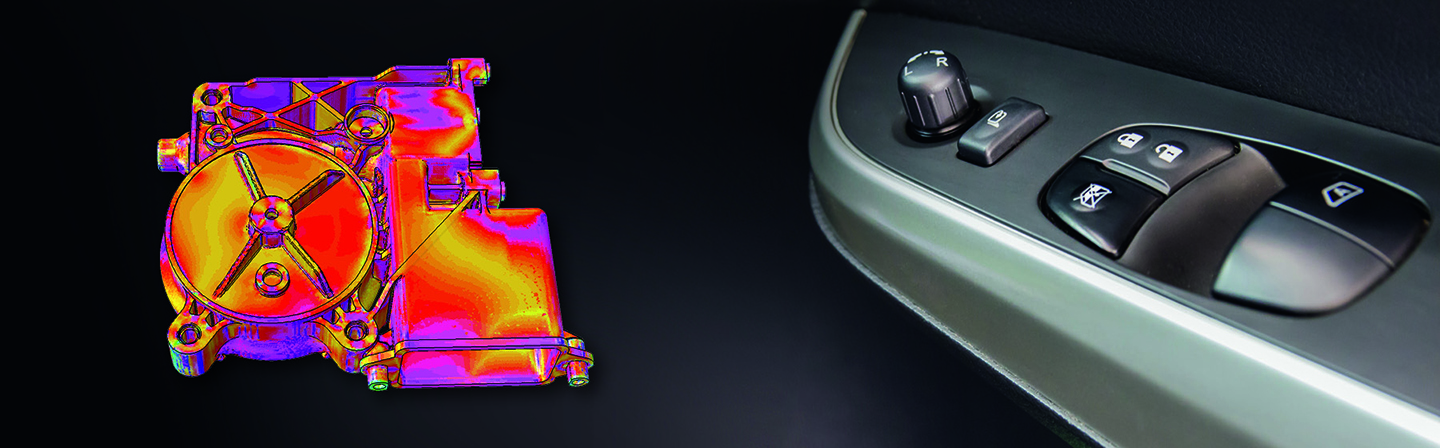
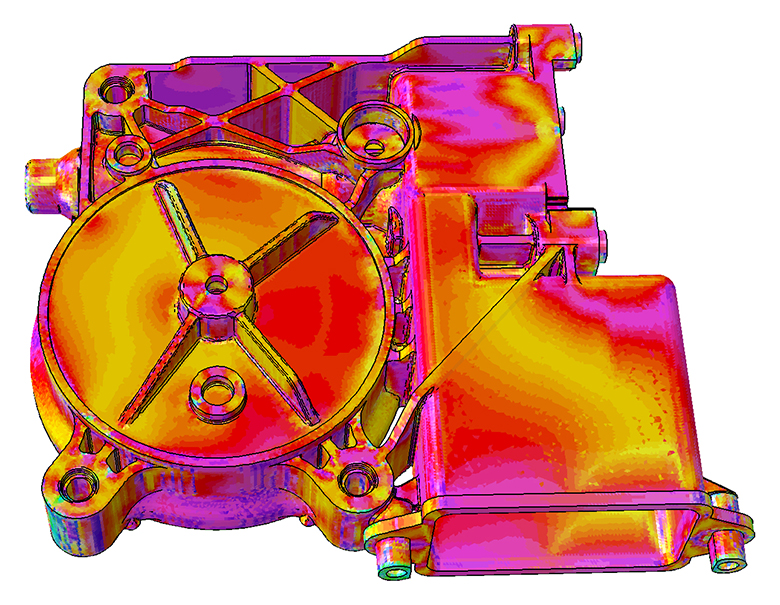
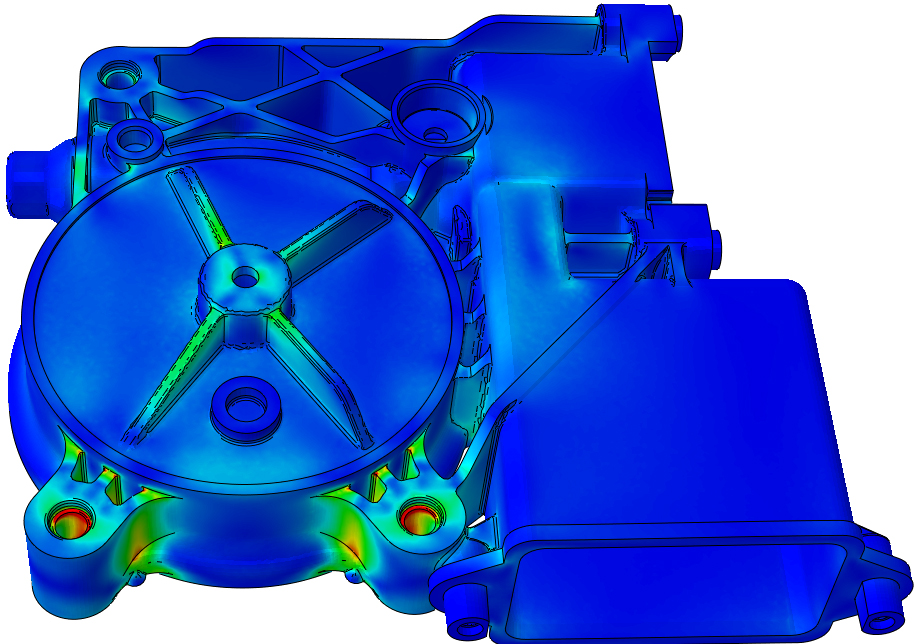
In industrial applications, components made of short fiber reinforced polymer composites (SFRP) are often subject to cyclic loads. In a cooperation with Bosch, we developed a multiscale simulation method to provide insight into the viscoelastic and fatigue behaviour of these components.
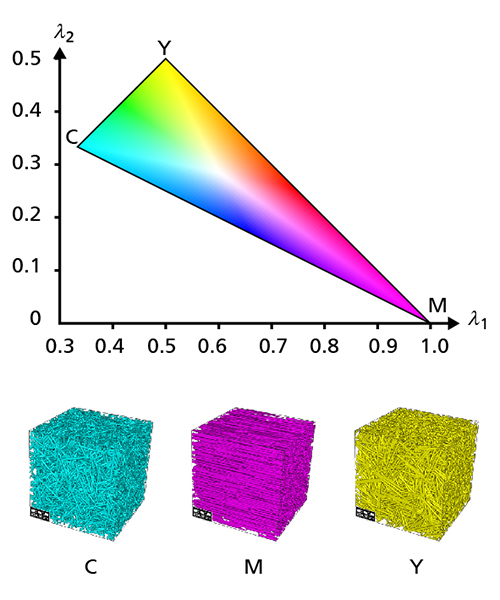
The elastic and non-linear material properties of injection molded parts strongly depend on the local fiber orientation, which varies continuously within the part: Due to the high length-diameter ratio of the fibers and the large difference between the macroscale of the component and microscale, resolving individual fibers is not possible. To overcome this issue and capture the interaction between the microstructure and macroscopic behavior we used a coupled FEM-FFT two-scale method.